Paul Zorn, St. Olaf College
Part II: Mathematics in Literature
Part III: What Is Mathematics?
Part IV: Mathematical Language: Learning from Barbie
Part V: Rule Books and Tour Guides: Two Live Questions
Part VI: Lessons from History
Pedagogical writing is certainly hard work. But it’s an honorable profession, and has been so for a long time. I’d like to cite, as evidence, two historical examples in point: Augustus de Morgan, who wrote actual textbooks, and Abraham de Moivre, who wrote in what seems to me a notably pedagogical style.
Consider first the British logician Augustus de Morgan (1806-71), whom we probably know best for his famous rules for complementing of unions and intersections.
De Morgan and I were both born in the South Indian state now called Tamilnadu; alas, the similarity ends there. In particular, de Morgan seems to have been a true prodigy, and was appointed to a mathematical chair at London University in 1827, at age 21, without any mathematical publications, expository or otherwise, to his credit.
But de Morgan became terrifically prolific, eventually publishing over 700 articles in something called the Penny Cyclopaedia, an outlet of the Society for the Diffusion of Useful Knowledge. (Victorian language aside, our beloved MAA and AMS are of course themselves devoted to slightly different aspects of that same high mission.)
Indisputably in the Useful Knowledge category was a Penny Cyclopaedia article called “Induction”, in which de Morgan carefully defined and described a process that had long, as one source puts it, “been used without clarity”. To recognize such a need and seek to fill it seems to me a poster-child example of mathematical exposition, also appropriately known as “the diffusion of useful knowledge”, at its very best.
One thing I admire especially about de Morgan is his continuing interest in education and its associated exposition. In a work called On the Study and Difficulties of Mathematics, de Morgan, then aged just 25, prescribed various remedies for what he saw as deficiencies in then-standard education in algebra. He describes the work as a sort of refresher course.
In the preface de Morgan argues, correctly but perhaps with some false humility, that more exalted persons of science should concern themselves with pedagogy and exposition.
The number of mathematical students, increased as it has been of late years, would be much augmented if those who hold the highest rank in science would condescend to give more effective assistance in clearing the elements of the difficulties which they present. If any one claiming that title should think my attempt obscure or erroneous, he must share the blame with me, since it is through his neglect that I have been enabled to avail myself of an opportunity to perform a task which I would gladly have seen confided to more skilful hands.
De Morgan was as good as his word. In his 30’s—and having indeed attained “the highest rank in science”—de Morgan published his Differential and Integral Calculus, but originally as a serial in 25 parts, each of 32 pages. (One might note, by the way, that this adds up to 800 pages. Complaints about overlong calculus texts may still be valid, but they go back a long way.)
Like all good textbook authors, de Morgan had a particular point of view, emphasizing limits, as opposed to the Lagrangian approach, emphasizing series, which he takes several opportunities to disparage.
De Morgan’s notion of “function” wasn’t quite the modern one; he means something like what we’d call a piecewise algebraic function, and he derives a correspondingly strong result:
Every function has a finite [derivative] when x has the specific value a, or when it has a value a+k, where k may be as small as we please.
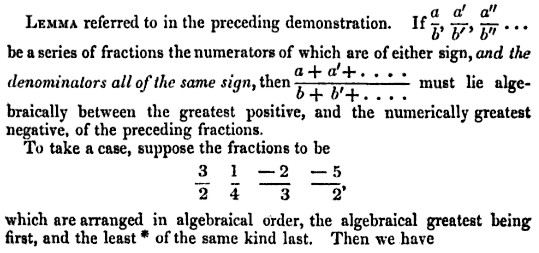
But de Morgan gives a clear and discursive proof, based on a clever lemma related to Farey fractions, which he illustrates with a simple numerical example.
This strikes me as good pedagogical writing, with useful asides to the reader and generously seasoned with concrete examples.
Concrete examples are sometimes denigrated as useless padding in modern textbooks, and perhaps there’s a point of overkill. But it’s worth noting that using concrete examples to illustrate general ideas and methods has a long history among great mathematicians.
Abraham de Moivre (1667-1754), for example, was a contemporary, friend, and in some respects a colleague, though a relatively luckless one, of Isaac Newton. He dedicated his most famous work, The Doctrine of Chances, to Newton. Newton must have respected de Moivre, referring questions on the Principia to him. “Go to de Moivre,” Newton is supposed to have said. “He knows these things better than I do.”
I mention de Moivre because I’m struck by his down-to-earth expository style, rich with concrete examples and special cases. Here’s an example: